|
|
|
\require{AMSmath}



Poisson van binomiaal
Ik ben bezig met een praktische opdracht over de poisson verdeling en heb een site in het Engels gevonden op deze site, ik begreep er iets meer van alleen ik snapte iets niet dat in het engels stond in het volgende stukje;
The main difference in the conditions between Binomial and Poisson was that with Poisson p
was unknown but small. If p is known, but small we are also allowed to use a Poisson
approximation. p should be smaller than 0.1 or in other words: p 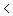 0.1 0.1
The main difference in the tables was that binomial uses p, but Poisson uses the mean. In
order to change the Binomial situation in a Poisson situation we need a mean. The rule is:
Mean = np
The reason for this approximation is because the Binomial cumulative table starts with
p = 0.1. For smaller values there is nothing.
In conclusion:
If p 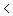 0.1 and you are using discrete data, use Poisson approximation with mean = np 0.1 and you are using discrete data, use Poisson approximation with mean = np
wat wordt er bedoeld met mean = np?
Alvast bedankt!
maartj
Leerling bovenbouw havo-vwo - donderdag 22 februari 2007
Antwoord
Hier wordt gezegd dat je een binomiale verdeling mag benaderen door een Poissonverdeling wanneer p 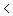 0,1. Dat is echter erg kort door de bocht. Zie ook mijn andere antwoord. 0,1. Dat is echter erg kort door de bocht. Zie ook mijn andere antwoord.
Die mean=nĚp slaat op de verwachtingswaarde van een binomiale verdeling en ook van de Poissonverdeling. Zelf even uitzoeken hoe dat zit.
Met vriendelijke groet
JaDeX

|
Vragen naar aanleiding van dit antwoord? Klik rechts..!
zaterdag 24 februari 2007
|

|



home |
vandaag |
bijzonder |
gastenboek |
statistieken |
wie is wie? |
verhalen |
colofon
©2001-2024 WisFaq - versie 3
|








 0.1
0.1
