Topologie

Ik zit met een vraag die ik begrijp maar ik heb moeite om het antwoord netjes op te schrijven.

De vraag luidt: Prove that if S is bounded, it's closure is bounded.

Mijn antwoord is: Let S be a subset of . If S is closed, then bd S (the collection of all boundary points) is a subset of S. And if a set is closed, it contains not only it's interior points but also all of its accumulation points. Then of course the closure of S is bounded.

Ik weet niet oe ik het anders moet zeggen! het is zo triviaal.....

Maarte
Student universiteit - maandag 25 februari 2008

Antwoord

Je antwoord heeft bijna niets met de vraag te maken. Een verzameling S is `bounded' (=begrensd) als er een getal M bestaat zo dat |x|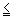M voor alle x in S. Als gegeven is dat S begrensd is moet je uit de M een getal N maken zo dat |x|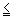N voor alle x in de afsluiting van S.
Hint: er geldt dat S een deelverzameling van het gesloten interval [-M,M] is; toon aan dat de afsluiting van S ook in dat interval zit.

kphart
maandag 25 februari 2008

©2001-2024 WisFaq