WisFaq!

Re: Re: Vergelijking oplossen

Okee, hoe moet ik deze vraag dan aanpakken? De vraag is dus:
Amanda assembles computers at her workstation. On average there are 47 computers in the system (waiting plus being assembled by Amanda). Assume that the number of arriving computers is distributed according to a Poisson distribution and that the time Amanda needs for assembling a computer is negative exponentially distributed. What is the probability that the number of computers in the system is less than or equal to 41 at an arbitrary point in time?

Ls (aantal in systeem) is dan 47.

Ze vragen de kans dat er minder of gelijk aan 41 zijn, dit is dus 1 - P meer dan 41.

Dus 1 - P (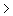41)

P = (Labda / Mu)^ (41+1)

en LS = Labda / (Mu - Labda) = 47

Hier loop ik dus vast, hoe kan ik dit het beste verder oplossen?

Martin
24-1-2009

Antwoord

Martin,
Poisson verdeling heeft parameter l en exp.verdeling heeft parameter m.Stel r=l/m.Als L=aantal computers in het systeem, dan is
E(L)=r/(1-r)= 47, dus r=47/48.Als P(n)=kans dat n computers in het systeem zitten,dan is P(n)=(1-r)r^n,n=0,1,2,...Verder moet het wel lukken.

kn
25-1-2009