WisFaq!

Re: Re: Bepalen van y tot de n-de, n element van N

Zit zo op de juiste koers? Gegeven: sin y = cos 2x

cos(pi/2-y)=cos2x; F(x,y)= cos2x-cos(pi/2-y)=0
d/dx F(x,y)=d/dx{cos2x-cos(pi/2-y)}=d/dx 0 --
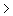
-2sin2x + sin(pi/2-y)y'= 0 -- 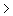y'= 2sin(2x)/sin(pi/2-y)--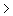
Nu op weg naar y"
d/dx(-2sin2x + sin(pi/2-y)y') = 0 --
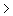
-4cos2x + cos(pi/2-y)y'+ sin(pi/2-y)y"= 0 -- 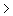Nu y' substitueren!
-4cos2x + cos(pi/2-y)(2sin2x/sin(pi/2-y)+ sin(pi/2-y)y"=0
Hieruit y" isoleren enz?

Johan uit de Bos
6-1-2009

Antwoord

Zo kan je bezig blijven, eigenlijk wordt het zo een cirkelredenering, wat volgens mij niet de bedoeling is.

Probeer eens y expliciet als functie van x te schrijven, dus y=f(x). (meerdere mogelijkheden). Die kan je vervolgens makkelijk differentiëren.

Bernhard
6-1-2009