WisFaq!

Re: Maple: complexe oplossingen

Ok bedankt voor het snelle antwoord, maar kom er nu achter dat ik

2z^4 + 9z +1 = 0

had moeten gebruiken! Nu heb ik gekeken en er komt uit als ik:

solve(2*z^4 + 9*z + 1 = 0, z);

RootOf(2*_Z^4+9*_Z+1, index = 1), RootOf(2*_Z^4+9*_Z+1, index = 2), RootOf(2*_Z^4+9*_Z+1, index = 3), RootOf(2*_Z^4+9*_Z+1, index = 4)

is dit een complex getal??

Albert de Vee
18-10-2004

Antwoord

In de 'handleiding' (Help) van Maple vind je:

- The function RootOf is a place holder for representing all the roots of an equation in one variable. In particular, it is the standard representation for Maple's algebraic numbers, algebraic functions (see evala), and finite fields GF(p^k), p prime, k 1 (see mod).
- Maple automatically generates RootOfs to express the solutions to polynomial equations and systems of equations (see solve), eigenvalues, and rational function integrals (see int). Maple knows how to apply diff, series, evalf, and simplify to RootOf expressions.

Als je na de laatste opdracht (die met het antwoord RootOf) de volgende opdracht geeft:

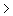evalf(%);

dan geeft Maple:

.8616365167+1.431108821*I, -.1111450227, -1.612128011, .8616365167-1.431108821*I

Dus twee reële oplossingen, en twee (toegevoegd) complexe.

dk
18-10-2004